As The Pressure Of A System Changes, Boiling Points Can Change In What Direction?

11.4 Vapor Pressure

Learning Objective

1. To know how and why the vapor pressure of a liquid varies with temperature.

Most all of us accept heated a pan of water with the hat in place and shortly thereafter heard the sounds of the lid rattling and hot water spilling onto the stovetop. When a liquid is heated, its molecules obtain sufficient kinetic energy to overcome the forces holding them in the liquid and they escape into the gaseous phase. By doing so, they generate a population of molecules in the vapor stage to a higher place the liquid that produces a pressure—the vapor pressureThe pressure created over a liquid by the molecules of a liquid substance that have enough kinetic free energy to escape to the vapor phase. of the liquid. In the situation nosotros described, enough pressure was generated to move the lid, which allowed the vapor to escape. If the vapor is independent in a sealed vessel, however, such as an unvented flask, and the vapor pressure level becomes too high, the flask will explode (every bit many students have unfortunately discovered). In this section, we describe vapor pressure level in more detail and explain how to quantitatively decide the vapor pressure level of a liquid.

Evaporation and Condensation

Considering the molecules of a liquid are in abiding motion, nosotros can plot the fraction of molecules with a given kinetic free energy (KE) against their kinetic energy to obtain the kinetic free energy distribution of the molecules in the liquid (Figure eleven.xiii "The Distribution of the Kinetic Energies of the Molecules of a Liquid at Ii Temperatures"), simply as we did for a gas (Figure 10.nineteen "The Wide Variation in Molecular Speeds Observed at 298 K for Gases with Different Molar Masses"). Every bit for gases, increasing the temperature increases both the average kinetic energy of the particles in a liquid and the range of kinetic energy of the individual molecules. If we assume that a minimum amount of energy (Due east ₀) is needed to overcome the intermolecular bonny forces that hold a liquid together, then some fraction of molecules in the liquid always has a kinetic free energy greater than E ₀. The fraction of molecules with a kinetic energy greater than this minimum value increases with increasing temperature. Whatever molecule with a kinetic energy greater than Eastward ₀ has plenty energy to overcome the forces holding it in the liquid and escape into the vapor phase. Before it can do so, notwithstanding, a molecule must as well be at the surface of the liquid, where it is physically possible for it to leave the liquid surface; that is, only molecules at the surface can undergo evaporation (or vaporization)The physical process by which atoms or molecules in the liquid phase enter the gas or vapor phase. , where molecules gain sufficient energy to enter a gaseous land above a liquid's surface, thereby creating a vapor pressure.

Figure 11.thirteen The Distribution of the Kinetic Energies of the Molecules of a Liquid at Two Temperatures

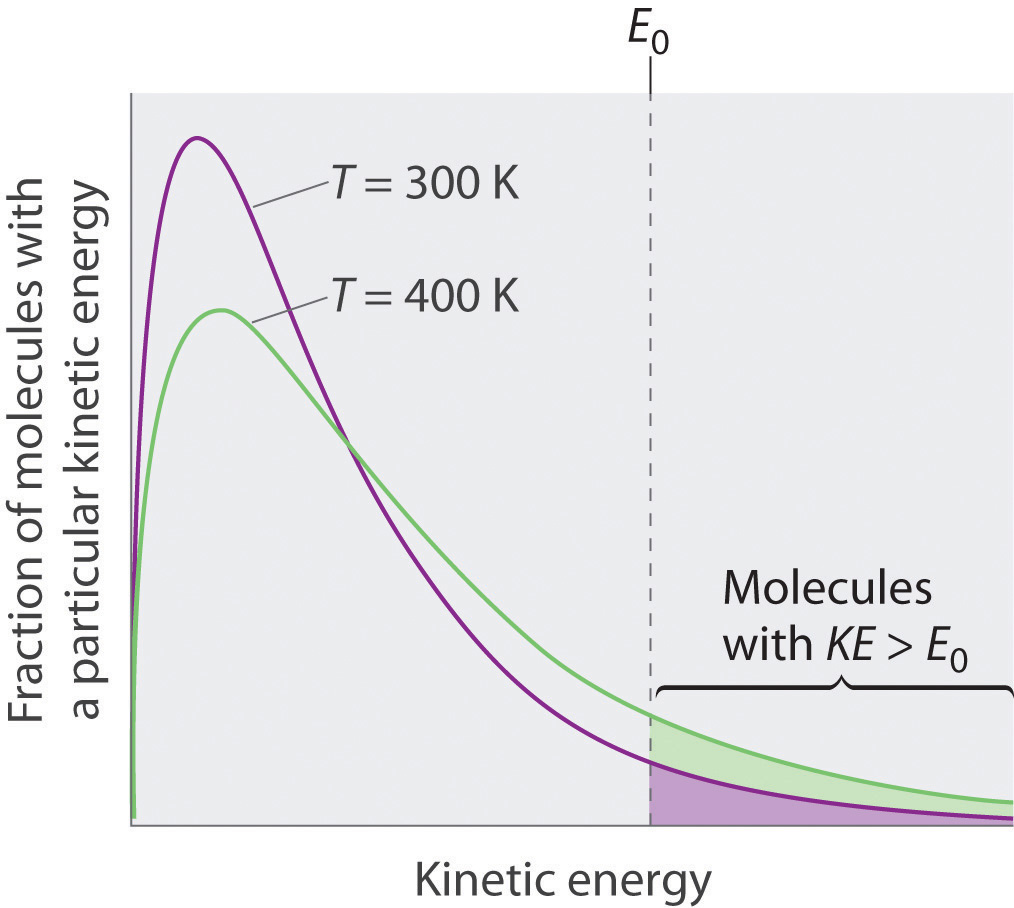
Just as with gases, increasing the temperature shifts the superlative to a college energy and broadens the curve. Only molecules with a kinetic free energy greater than Eastward ₀ can escape from the liquid to enter the vapor phase, and the proportion of molecules with KE >Due east ₀ is greater at the higher temperature.

To understand the causes of vapor pressure, consider the apparatus shown in Effigy 11.14 "Vapor Pressure". When a liquid is introduced into an evacuated chamber (function (a) in Effigy 11.14 "Vapor Pressure"), the initial pressure level above the liquid is approximately zero because there are as yet no molecules in the vapor stage. Some molecules at the surface, however, will have sufficient kinetic free energy to escape from the liquid and course a vapor, thus increasing the pressure inside the container. As long equally the temperature of the liquid is held constant, the fraction of molecules with KE >East ₀ will not modify, and the rate at which molecules escape from the liquid into the vapor phase will depend only on the surface area of the liquid phase.

Figure eleven.fourteen Vapor Pressure

(a) When a liquid is introduced into an evacuated bedroom, molecules with sufficient kinetic energy escape from the surface and enter the vapor phase, causing the pressure in the sleeping room to increase. (b) When sufficient molecules are in the vapor stage for a given temperature, the rate of condensation equals the rate of evaporation (a steady land is reached), and the pressure in the container becomes abiding.

Every bit soon every bit some vapor has formed, a fraction of the molecules in the vapor phase volition collide with the surface of the liquid and reenter the liquid stage in a process known every bit condensationThe concrete process by which atoms or molecules in the vapor phase enter the liquid phase. (role (b) in Figure 11.14 "Vapor Force per unit area"). Every bit the number of molecules in the vapor phase increases, the number of collisions between vapor-stage molecules and the surface will also increase. Eventually, a steady land will exist reached in which exactly as many molecules per unit time leave the surface of the liquid (vaporize) as collide with it (condense). At this bespeak, the pressure level over the liquid stops increasing and remains constant at a particular value that is characteristic of the liquid at a given temperature. The rates of evaporation and condensation over fourth dimension for a system such as this are shown graphically in Figure 11.xv "The Relative Rates of Evaporation and Condensation as a Function of Time after a Liquid Is Introduced into a Sealed Sleeping room".

Figure eleven.fifteen The Relative Rates of Evaporation and Condensation as a Function of Fourth dimension after a Liquid Is Introduced into a Sealed Chamber

The rate of evaporation depends only on the surface surface area of the liquid and is essentially abiding. The charge per unit of condensation depends on the number of molecules in the vapor stage and increases steadily until information technology equals the rate of evaporation.

Equilibrium Vapor Force per unit area

2 opposing processes (such as evaporation and condensation) that occur at the aforementioned rate and thus produce no internet change in a system, found a dynamic equilibriumA state in which two opposing processes occur at the same rate, thus producing no cyberspace alter in the organization. . In the instance of a liquid enclosed in a chamber, the molecules continuously evaporate and condense, only the amounts of liquid and vapor do not alter with time. The pressure exerted by a vapor in dynamic equilibrium with a liquid is the equilibrium vapor pressureThe force per unit area exerted by a vapor in dynamic equilibrium with its liquid. of the liquid.

If a liquid is in an open container, all the same, well-nigh of the molecules that escape into the vapor phase will not collide with the surface of the liquid and return to the liquid stage. Instead, they volition diffuse through the gas phase away from the container, and an equilibrium will never be established. Under these weather condition, the liquid will continue to evaporate until it has "disappeared." The speed with which this occurs depends on the vapor pressure of the liquid and the temperature. Volatile liquidsA liquid with a relatively high vapor force per unit area. have relatively high vapor pressures and tend to evaporate readily; nonvolatile liquidsA liquid with a relatively low vapor pressure. have low vapor pressures and evaporate more slowly. Although the dividing line between volatile and nonvolatile liquids is not articulate-cut, as a general guideline, nosotros can say that substances with vapor pressures greater than that of h2o (Table 11.four "Surface Tension, Viscosity, Vapor Pressure (at 25°C Unless Otherwise Indicated), and Normal Boiling Points of Common Liquids") are relatively volatile, whereas those with vapor pressures less than that of water are relatively nonvolatile. Thus diethyl ether (ethyl ether), acetone, and gasoline are volatile, only mercury, ethylene glycol, and motor oil are nonvolatile.

The equilibrium vapor force per unit area of a substance at a item temperature is a feature of the material, like its molecular mass, melting signal, and humid betoken (Tabular array 11.4 "Surface Tension, Viscosity, Vapor Pressure (at 25°C Unless Otherwise Indicated), and Normal Boiling Points of Common Liquids"). Information technology does not depend on the amount of liquid as long every bit at least a tiny amount of liquid is present in equilibrium with the vapor. The equilibrium vapor pressure level does, however, depend very strongly on the temperature and the intermolecular forces present, as shown for several substances in Figure 11.16 "The Vapor Pressures of Several Liquids as a Function of Temperature". Molecules that can hydrogen bail, such equally ethylene glycol, take a much lower equilibrium vapor force per unit area than those that cannot, such as octane. The nonlinear increment in vapor pressure level with increasing temperature is much steeper than the increase in pressure expected for an platonic gas over the corresponding temperature range. The temperature dependence is so strong considering the vapor force per unit area depends on the fraction of molecules that have a kinetic energy greater than that needed to escape from the liquid, and this fraction increases exponentially with temperature. As a consequence, sealed containers of volatile liquids are potential bombs if subjected to large increases in temperature. The gas tanks on automobiles are vented, for example, and then that a car won't explode when parked in the sun. Similarly, the small cans (1–v gallons) used to transport gasoline are required past law to take a popular-off force per unit area release.

Effigy 11.16 The Vapor Pressures of Several Liquids every bit a Function of Temperature

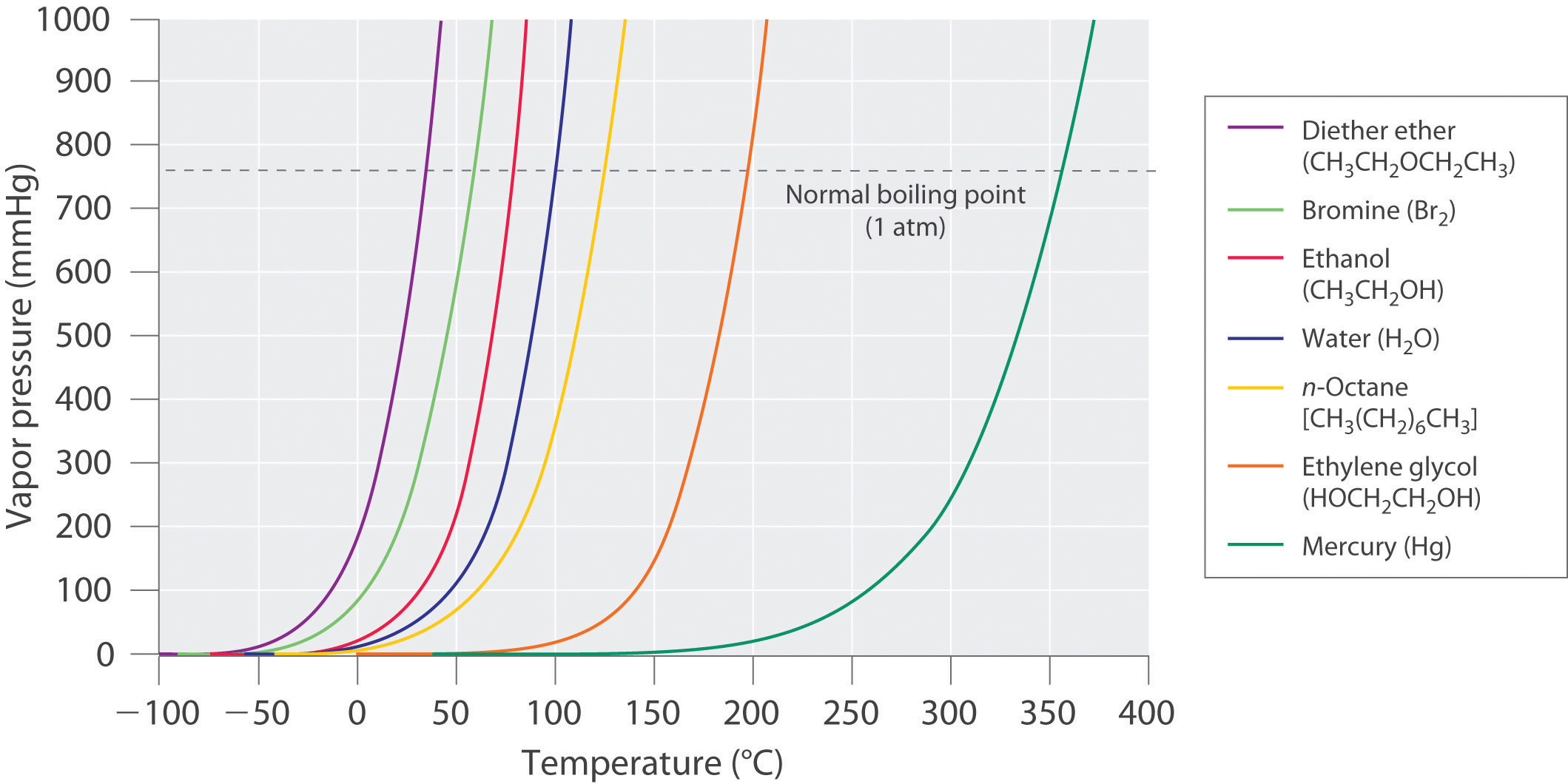
The betoken at which the vapor pressure level bend crosses the P = 1 atm line (dashed) is the normal boiling bespeak of the liquid.

Note the Pattern

Volatile substances have low boiling points and relatively weak intermolecular interactions; nonvolatile substances have high boiling points and relatively stiff intermolecular interactions.

The exponential ascension in vapor force per unit area with increasing temperature in Figure 11.16 "The Vapor Pressures of Several Liquids as a Role of Temperature" allows u.s. to use natural logarithms to express the nonlinear human relationship every bit a linear ane.For a review of natural logarithms, refer to Essential Skills 6 in Section eleven.9 "Essential Skills half-dozen".

Equation eleven.1

$$\begin{array}{cccccc}& \text{ln}P& =& \frac{-\Delta H_{\text{vap}}}{R}\left(\frac{1}{T}\right)& +& C\\ \text{Equation for a direct line}:& y& =& \mathrm{chiliad}x& +& \text{b}\end{array}$$

where ln P is the natural logarithm of the vapor pressure, Δ H _vap is the enthalpy of vaporization, R is the universal gas abiding [8.314 J/(mol·Grand)], T is the temperature in kelvins, and C is the y-intercept, which is a abiding for any given line. A plot of ln P versus the inverse of the accented temperature (ane/T) is a directly line with a gradient of −ΔH _vap/R. Equation xi.1, called the Clausius–Clapeyron equationA linear human relationship that expresses the nonlinear human relationship betwixt the vapor pressure of a liquid and temperature: ln $P=-\Delta H_{\text{vap}}/RT+C,$ where $P$ is pressure, $\Delta H_{\text{vap}}$ is the heat of vaporization, $R$ is the universal gas constant, $T$ is the absolute temperature, and C is a abiding. The Clausius–Clapeyron equation can be used to summate the heat of vaporization of a liquid from its measured vapor pressure at ii or more temperatures. , can exist used to calculate the ΔH _vap of a liquid from its measured vapor force per unit area at two or more than temperatures. The simplest mode to determine ΔH _vap is to mensurate the vapor pressure of a liquid at two temperatures and insert the values of P and T for these points into Equation xi.2, which is derived from the Clausius–Clapeyron equation:

Equation 11.2

$$\ln \left(\frac{P_2}{P_1}\right)=\frac{-\Delta H_{\mathrm{five}ap}}{R}\left(\frac{\mathrm{one}}{T_2}-\frac{1}{T_{\mathrm{i}}}\right)$$

Conversely, if we know ΔH _vap and the vapor pressure P ₁ at any temperature T ₁, nosotros can use Equation 11.ii to calculate the vapor force per unit area P _two at any other temperature T _two, as shown in Example 6.

Example 6

The experimentally measured vapor pressures of liquid Hg at 4 temperatures are listed in the post-obit table:

T (°C)	80.0	100	120	140
P (torr)	0.0888	0.2729	0.7457	ane.845

From these data, summate the enthalpy of vaporization (ΔH _vap) of mercury and predict the vapor force per unit area of the liquid at 160°C. (Safety note: mercury is highly toxic; when it is spilled, its vapor pressure generates hazardous levels of mercury vapor.)

Given: vapor pressures at four temperatures

Asked for: ΔH _vap of mercury and vapor pressure at 160°C

Strategy:

A Use Equation 11.two to obtain ΔH _vap straight from two pairs of values in the table, making certain to catechumen all values to the appropriate units.

B Substitute the calculated value of ΔH _vap into Equation 11.2 to obtain the unknown pressure (P ₂).

Solution:

A The tabular array gives the measured vapor pressures of liquid Hg for four temperatures. Although one way to proceed would be to plot the data using Equation 11.one and detect the value of ΔH _vap from the slope of the line, an alternative approach is to utilize Equation 11.ii to obtain ΔH _vap direct from two pairs of values listed in the table, assuming no errors in our measurement. We therefore select two sets of values from the table and convert the temperatures from degrees Celsius to kelvins because the equation requires absolute temperatures. Substituting the values measured at lxxx.0°C (T _i) and 120.0°C (T ₂) into Equation xi.two gives

$$\begin{array}{c}\ln \left(\frac{0.7457\cancel{\text{torr}}}{0.0888\cancel{\text{torr}}}\right)=\frac{-\Delta H_{\text{vap}}}{\mathrm{viii.314}\text{ J/(mol}·\text{K)}}\left[\frac{\mathrm{ane}}{(120+273)\text{Chiliad}}-\frac{\mathrm{ane}}{(80.0+273)\text{ Thou}}\right]\\ \ln (8.398)=\frac{-\Delta H_{\text{vap}}}{8.314\text{ J}·{\text{mol}}^{-1}·\cancel{{\text{G}}^{-1}}}\left(-\mathrm{ii.88}\times {\mathrm{x}}^{-\mathrm{four}}\cancel{{\text{K}}^{-1}}\right)\\ 2.13=-\Delta H_{\text{vap}}\left(-0.346\times {\mathrm{ten}}^{-\mathrm{four}}\right){\text{ J}}^{-1}·\text{mol}\\ \Delta H_{\text{vap}}=\mathrm{61,400}\text{ J/mol}=\text{61}\text{.iv kJ/mol}\end{array}$$

B We can at present use this value of ΔH _vap to calculate the vapor pressure of the liquid (P ₂) at 160.0°C (T ₂):

$$\ln \left(\frac{P_2}{0.0888\text{ torr}}\right)=\frac{-\mathrm{61,400}\cancel{\text{ J}}·\cancel{{\text{mol}}^{-\mathrm{one}}}}{8.314\cancel{\text{ J}}·\cancel{{\text{mol}}^{-\mathrm{one}}}·{\text{G}}^{-\mathrm{one}}}\left[\frac{1}{(160+273)\text{ 1000}}-\frac{\mathrm{one}}{(80.0+273)\text{ K}}\right]=3.86$$

Using the human relationship eastward ^{ln x}  =x, we have

$$\begin{array}{l}\ln \left(\frac{P_2}{0.0888\text{ torr}}\right)=3.86\\ \frac{P_2}{0.0888\text{ torr}}=e^{3.86}=\mathrm{47.five}\\ P_2=4.21\text{ torr}\end{array}$$

At 160°C, liquid Hg has a vapor force per unit area of four.21 torr, substantially greater than the pressure level at 80.0°C, equally we would expect.

Exercise

The vapor pressure of liquid nickel at 1606°C is 0.100 torr, whereas at 1805°C, its vapor pressure is one.000 torr. At what temperature does the liquid have a vapor pressure of two.500 torr?

Respond: 1896°C

Boiling Points

As the temperature of a liquid increases, the vapor pressure of the liquid increases until it equals the external pressure level, or the atmospheric force per unit area in the case of an open container. Bubbles of vapor begin to class throughout the liquid, and the liquid begins to boil. The temperature at which a liquid boils at exactly one atm pressure is the normal boiling pointThe temperature at which a substance boils at a pressure of i atm. of the liquid. For water, the normal boiling point is exactly 100°C. The normal boiling points of the other liquids in Figure 11.16 "The Vapor Pressures of Several Liquids as a Part of Temperature" are represented by the points at which the vapor pressure curves cantankerous the line corresponding to a pressure of 1 atm. Although we usually cite the normal humid point of a liquid, the bodily boiling indicate depends on the force per unit area. At a force per unit area greater than ane atm, water boils at a temperature greater than 100°C because the increased force per unit area forces vapor molecules higher up the surface to condense. Hence the molecules must have greater kinetic energy to escape from the surface. Conversely, at pressures less than 1 atm, h2o boils below 100°C.

Typical variations in atmospheric pressure at sea level are relatively small, causing only minor changes in the boiling betoken of water. For example, the highest recorded atmospheric pressure at bounding main level is 813 mmHg, recorded during a Siberian wintertime; the lowest sea-level pressure ever measured was 658 mmHg in a Pacific typhoon. At these pressures, the humid point of h2o changes minimally, to 102°C and 96°C, respectively. At high altitudes, on the other hand, the dependence of the boiling point of h2o on pressure becomes pregnant. Table 11.5 "The Boiling Points of Water at Various Locations on Earth" lists the boiling points of h2o at several locations with different altitudes. At an elevation of only 5000 ft, for example, the boiling point of water is already lower than the everyman ever recorded at ocean level. The lower boiling point of water has major consequences for cooking everything from soft-boiled eggs (a "3-minute egg" may well have four or more minutes in the Rockies and even longer in the Himalayas) to cakes (block mixes are often sold with separate high-altitude instructions). Conversely, pressure cookers, which have a seal that allows the pressure inside them to exceed ane atm, are used to melt food more apace by raising the humid point of water and thus the temperature at which the food is beingness cooked.

Note the Pattern

As force per unit area increases, the boiling point of a liquid increases and vice versa.

Table 11.5 The Humid Points of H2o at Various Locations on Globe

Identify	Altitude above Sea Level (ft)	Atmospheric Force per unit area (mmHg)	Boiling Point of Water (°C)
Mt. Everest, Nepal/Tibet	29,028	240	70
Bogota, Colombia	xi,490	495	88
Denver, Colorado	5280	633	95
Washington, DC	25	759	100
Dead Sea, State of israel/Hashemite kingdom of jordan	−1312	799	101.four

Example 7

Use Effigy 11.xvi "The Vapor Pressures of Several Liquids equally a Office of Temperature" to estimate the following.

1. the boiling betoken of water in a pressure cooker operating at 1000 mmHg
2. the pressure required for mercury to boil at 250°C

Given: data in Figure eleven.16 "The Vapor Pressures of Several Liquids as a Function of Temperature", pressure, and boiling point

Asked for: corresponding boiling point and pressure

Strategy:

A To estimate the humid betoken of water at m mmHg, refer to Effigy eleven.xvi "The Vapor Pressures of Several Liquids as a Part of Temperature" and discover the point where the vapor pressure level curve of h2o intersects the line corresponding to a pressure of m mmHg.

B To gauge the pressure required for mercury to boil at 250°C, observe the point where the vapor pressure curve of mercury intersects the line respective to a temperature of 250°C.

Solution:

1. A The vapor force per unit area bend of water intersects the P = 1000 mmHg line at about 110°C; this is therefore the boiling point of water at 1000 mmHg.
2. B The vertical line corresponding to 250°C intersects the vapor pressure bend of mercury at P ≈ 75 mmHg. Hence this is the pressure level required for mercury to boil at 250°C.

Exercise

Utilize the data in Figure xi.16 "The Vapor Pressures of Several Liquids as a Function of Temperature" to estimate the following.

1. the normal boiling point of ethylene glycol
2. the force per unit area required for diethyl ether to eddy at 20°C.

Reply:

1. 200°C
2. 450 mmHg

Summary

Because the molecules of a liquid are in constant motion and possess a wide range of kinetic energies, at any moment some fraction of them has enough free energy to escape from the surface of the liquid to enter the gas or vapor stage. This process, called vaporization or evaporation, generates a vapor pressure above the liquid. Molecules in the gas phase tin collide with the liquid surface and reenter the liquid via condensation. Eventually, a steady land is reached in which the number of molecules evaporating and condensing per unit time is the same, and the system is in a state of dynamic equilibrium. Under these conditions, a liquid exhibits a characteristic equilibrium vapor pressure that depends only on the temperature. Nosotros can limited the nonlinear relationship between vapor force per unit area and temperature as a linear relationship using the Clausius–Clapeyron equation. This equation tin can be used to summate the enthalpy of vaporization of a liquid from its measured vapor pressure at ii or more temperatures. Volatile liquids are liquids with high vapor pressures, which tend to evaporate readily from an open container; nonvolatile liquids have low vapor pressures. When the vapor pressure equals the external pressure, bubbles of vapor grade inside the liquid, and it boils. The temperature at which a substance boils at a pressure level of 1 atm is its normal boiling signal.

Key Takeaways

• The equilibrium vapor force per unit area of a liquid depends on the temperature and the intermolecular forces present.
• The human relationship betwixt pressure, enthalpy of vaporization, and temperature is given by the Clausius-Clapeyron equation.

Key Equations

Clausius–Clapeyron equation

Equation 11.1: $\text{ln}P=\frac{-\Delta H_{\text{vap}}}{R}\left(\frac{1}{T}\right)+C$

Using vapor pressure at ii temperatures to calculate Δ H _vap

Equation 11.ii: $\text{ln}\left(\frac{P_2}{P_1}\right)=\frac{-\Delta H_{\text{vap}}}{R}\left(\frac{1}{T_{\mathrm{two}}}-\frac{\mathrm{ane}}{T_1}\right)$

Conceptual Problems

1. What is the relationship between the boiling signal, vapor force per unit area, and temperature of a substance and atmospheric pressure?

2. What is the difference between a volatile liquid and a nonvolatile liquid? Suppose that two liquid substances take the aforementioned molecular mass, simply ane is volatile and the other is nonvolatile. What differences in the molecular structures of the two substances could account for the differences in volatility?

3. An "old wives' tale" states that applying ethanol to the wrists of a child with a very high fever will assistance to reduce the fever considering blood vessels in the wrists are close to the skin. Is in that location a scientific footing for this recommendation? Would water be every bit effective as ethanol?

4. Why is the air over a strip of grass significantly cooler than the air over a sandy beach only a few feet away?

5. If gasoline is allowed to sit down in an open container, it often feels much colder than the surrounding air. Explain this observation. Draw the flow of heat into or out of the system, as well every bit whatever transfer of mass that occurs. Would the temperature of a sealed can of gasoline exist higher, lower, or the aforementioned as that of the open up can? Explain your reply.

6. What is the relationship between the vapor pressure of a liquid and

   1. its temperature?
   2. the surface expanse of the liquid?
   3. the pressure of other gases on the liquid?
   4. its viscosity?

7. At 25°C, benzene has a vapor force per unit area of 12.5 kPa, whereas the vapor pressure of acetic acid is 2.1 kPa. Which is more than volatile? Based on the intermolecular interactions in the two liquids, explain why acetic acid has the lower vapor pressure level.

Numerical Issues

1. Acetylene (C_twoH_ii), which is used for industrial welding, is transported in pressurized cylinders. Its vapor pressure at various temperatures is given in the following tabular array. Plot the data and use your graph to estimate the vapor pressure of acetylene at 293 K. Then use your graph to decide the value of ΔH _vap for acetylene. How much energy is required to vaporize 2.00 g of acetylene at 250 K?

   T (Thousand)	145	155	175	200	225	250	300
   P (mmHg)	ane.3	7.8	32.2	190	579	1370	5093

2. The following tabular array gives the vapor pressure of water at various temperatures. Plot the data and employ your graph to estimate the vapor pressure of water at 25°C and at 75°C. What is the vapor force per unit area of water at 110°C? Use these information to determine the value of ΔH _vap for water.

   T (°C)	0	10	thirty	50	60	80	100
   P (mmHg)	4.6	9.2	31.8	92.6	150	355	760

3. The ΔH _vap of carbon tetrachloride is 29.eight kJ/mol, and its normal humid point is 76.8°C. What is its boiling signal at 0.100 atm?

4. The normal boiling point of sodium is 883°C. If ΔH _vap is 97.iv kJ/mol, what is the vapor pressure (in millimeters of mercury) of liquid sodium at 300°C?

7. If the vapor force per unit area of a liquid is 0.850 atm at 20°C and 0.897 atm at 25°C, what is the normal boiling point of the liquid?

8. If the vapor force per unit area of a liquid is 0.799 atm at 99.0°C and 0.842 atm at 111°C, what is the normal boiling signal of the liquid?

9. The vapor pressure of liquid SO₂ is 33.iv torr at −63.iv°C and 100.0 torr at −47.vii K.

   1. What is the ΔH _vap of And then₂?
   2. What is its vapor pressure at −24.five One thousand?
   3. At what temperature is the vapor pressure level equal to 220 torr?

10. The vapor pressure of CO_ii at various temperatures is given in the post-obit table:

    T (°C)	−120	−110	−100	−xc
    P (torr)	ix.81	34.63	104.81	279.5

    1. What is ΔH _vap over this temperature range?
    2. What is the vapor pressure of CO₂ at −seventy°C?
    3. At what temperature does CO₂ have a vapor pressure of 310 torr?

Answers

1. 

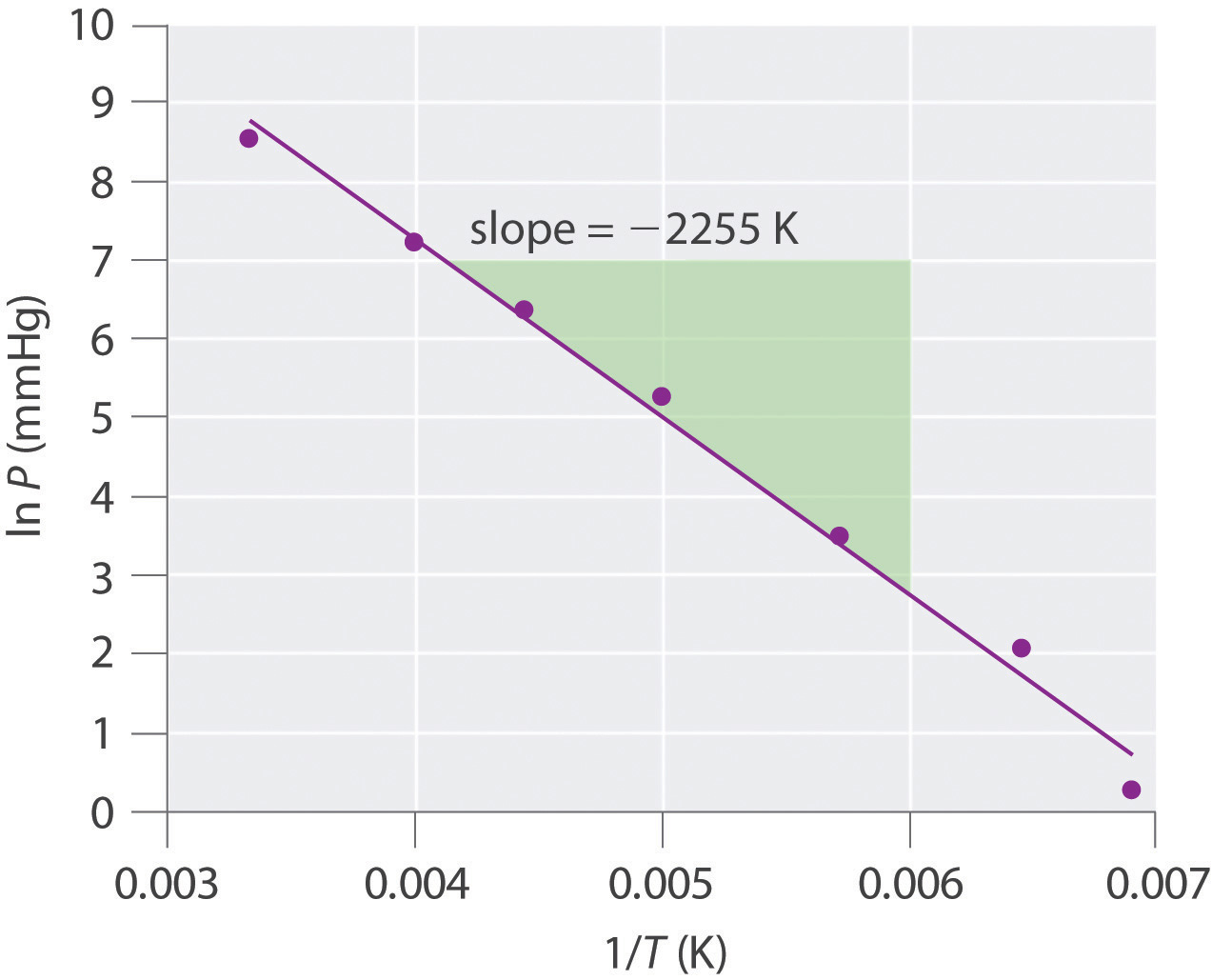
   vapor pressure at 273 Thou is 3050 mmHg; ΔH _vap = 18.7 kJ/mol, 1.44 kJ

3. 12.5°C

5. ΔH _vap = 28.nine kJ/mol, due north-hexane

7. ΔH _vap = vii.81 kJ/mol, 36°C

Source: https://saylordotorg.github.io/text_general-chemistry-principles-patterns-and-applications-v1.0/s15-04-vapor-pressure.html

Posted by: suttonthereatend.blogspot.com

Share this post